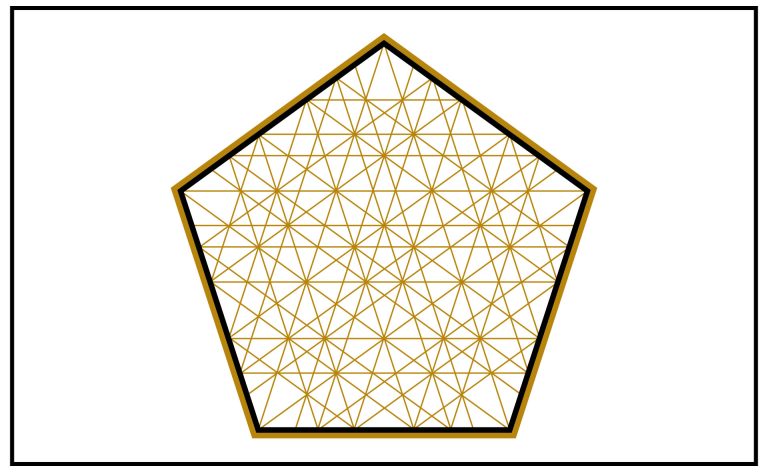
Forging The Golden Pentagon
In this article we will be looking at how the simple regular pentagon is in fact recursively resonant with Φ (Phi), the Golden Ratio. This article

In this article we will be looking at how the simple regular pentagon is in fact recursively resonant with Φ (Phi), the Golden Ratio. This article

In this article we will be looking at the use of Φ(Phi), or rather a very specific approximation of it, in the construction of this
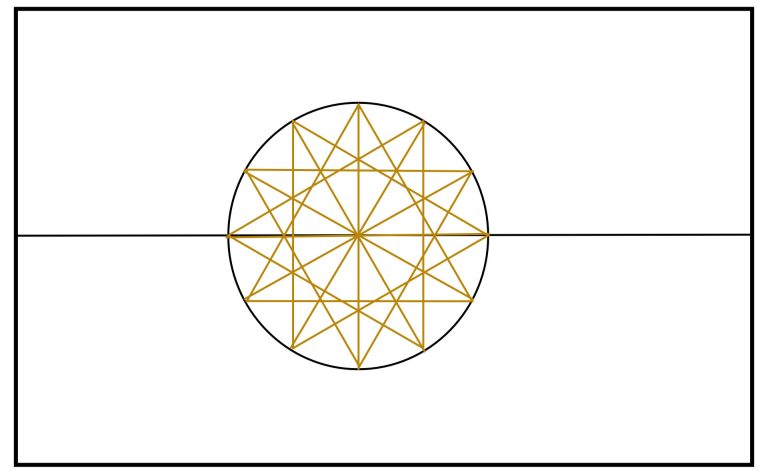
A Dodecagram (12-pointed star) is a simple and useful star to forge. From it you can build the circle of 12, which is used in
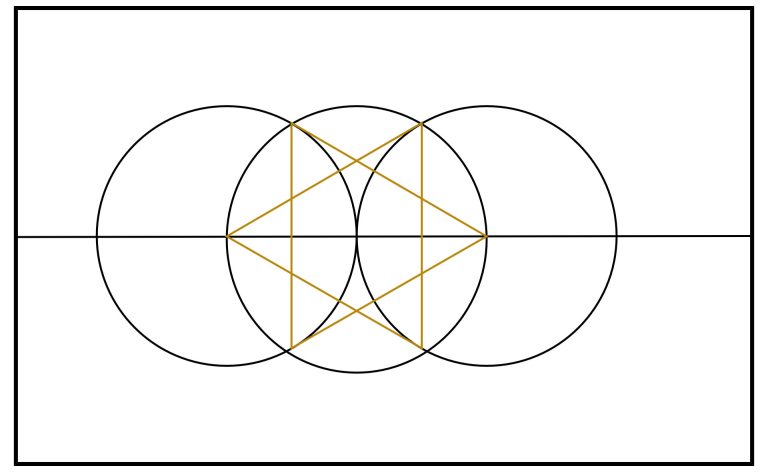
There are many ways to draw a hexagram. It is a very useful shape for splitting a circle up into 12. This can be used
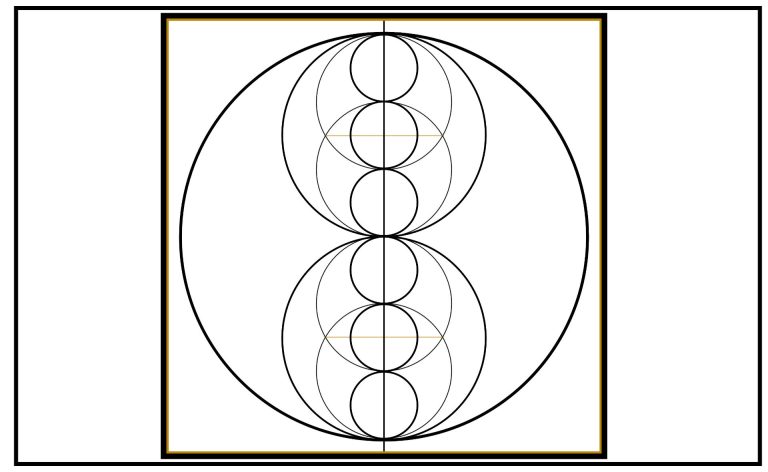
The Taijitu (Yin-Yang) is a simple yet profound Taoist symbol. We will discuss this in later articles. In this article we will show you how
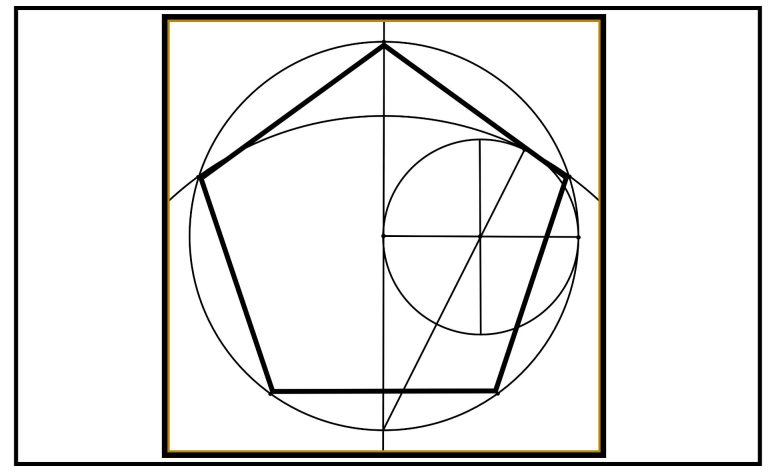
Whilst we have seen that we can create a pentagon from within the Golden Triangle, it is sometimes convenient to create a pentagon from scratch.

So, we finished off with the Golden Triangle an earlier article. In this we will continue to explore the self-similar properties of this triangle in

Now that we have the Golden Rectangle to hand, we can start exploring other geometric shapes with similar properties. One of the most obvious examples
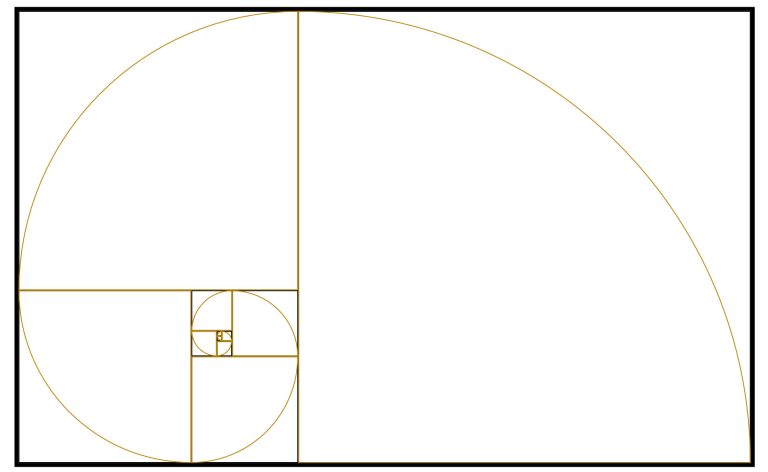
Now that we have the Golden Rectangle to hand it is trivial to create an approximation of the Golden Spiral. This can be done by

To construct and understand the Golden Rectangle it is necessary to understand a few basic concepts. These are the Fibonacci sequence and Φ (Phi), also known as the